Class -IX Unit-4 Linear equations in two variables
LINEAR EQUATION IN TWO VARIABLES
An equation of the form ax + by + c = 0 where a, b, c are real numbers and x, y are variables, is
called a linear equation in two variables.
Here ‘a’ is called coefficient of x, ‘b’ is called coefficients of y and c is called constant term.
Eg. 6x + 2y + 5 = 0, 5x – 2y + 3 = 0 etc.
SOLUTIONS OF LINEAR EQUATION
The value of the variable which when substituted for the variable in the equation satisfies the
equation i.e. L.H.S. and R.H.S. of the equation becomes equal, is called the solution or root of
the equation.
RULES FOR SOLVING AN EQUATION
(i) Same quantity can be added to both sides of an equation without changing the equality.
(ii) Same quantity can be subtracted from both sides of an equation without changing the
equality.
(iii) Both sides of an equation may be multiplied by a same non-zero number without
changing the equality.
(iv) Both sides of an equation may be divided by a same non-zero number without changing
the equality.
A LINEAR EQUATION EN TWO VARIABLES
HAS INFINITELY MANY SOLUTIONS
We can get many many solutions in the following way.
Pick a value of your choice for x (say x = 2) in 2x + 3y = 12. then the equation reduces to 4+3y
= 12, which is a linear equation in one variable. On solving this, you get y = 8/3. So (2, 8/3) is
another solution of 2x + 3y = 12. Similarly, choosing x = —5, you find that the equation
becomes — 10 + 3y = 12. This gives y =22/3. So, (-5, 22/3) is another solution of 2x + 3y = 12.
So there is no end to different solutions of a linear equation in two variables.
Note : An easy way of getting a solution is to take x = 0 and get the corresponding value of y.
Similarly, we can put y =0 and obtain the corresponding value of x.
GRAPH OF LINEAR EQUATIONS
The graph of an equation in x and y is the set of all points whose coordinates satisfy the
equation :
In order to draw the graph of a linear equation ax + by + c = 0 may follow the following
algorithm.
Step 1: Obtain the linear equation ax + by+ c = 0
Step 2 : Express yin terms of x i.e. y = -((ax + b)/c))
Step 3 : Put any two or three values for x and calculate the corresponding values of y from the
expression values of y from the expression obtained in step 2. Let we get points as (α1, β1), (α2,
β2), (α3, β3)
Step 4 : Plot points (α1, β1), (α2, β2), (α3, β3) on graph paper.
Step 5 : Join the points marked in step 4 to obtain. The line obtained is the graph of the
equation ax+by+c= 0
Note : (i) The reason that a degree one polynomial equation ax + by + c = 0 is called a linear
equation is that its geometrical representation is a straight line.
(ii) The graph of the equation of the form y = kx is a line which always passes through the
origin.
EQUATIONS OF LINES PARALLEL TO THE X— AXIS AND Y— AXIS
Every point on the x – axis is of the form (x, 0). The equation of the x – axis is given byy 0.
Note that y =0 can be expressed as 0. x + 1.)) 0.
Similarly, observe that the equation of they – axis is given by x = 0.
Consider the equation x -2 = 0. If this is treated as an equation in one variable x only, then it
has the unique solution x= 2, which is a point on the number line.
An equation in two variables, x -2 = 0 is represented by the line AB in the graph in below given
Note : (i) The graph ofx = a is a straight line parallel to they – axis
(ii) The graph ofy = a is a straight line parallel to thex – axis.
An equation of the form ax + by + c = 0 where a, b, c are real numbers and x, y are variables, is
called a linear equation in two variables.
Here ‘a’ is called coefficient of x, ‘b’ is called coefficients of y and c is called constant term.
Eg. 6x + 2y + 5 = 0, 5x – 2y + 3 = 0 etc.
SOLUTIONS OF LINEAR EQUATION
The value of the variable which when substituted for the variable in the equation satisfies the
equation i.e. L.H.S. and R.H.S. of the equation becomes equal, is called the solution or root of
the equation.
RULES FOR SOLVING AN EQUATION
(i) Same quantity can be added to both sides of an equation without changing the equality.
(ii) Same quantity can be subtracted from both sides of an equation without changing the
equality.
(iii) Both sides of an equation may be multiplied by a same non-zero number without
changing the equality.
(iv) Both sides of an equation may be divided by a same non-zero number without changing
the equality.
A LINEAR EQUATION EN TWO VARIABLES
HAS INFINITELY MANY SOLUTIONS
We can get many many solutions in the following way.
Pick a value of your choice for x (say x = 2) in 2x + 3y = 12. then the equation reduces to 4+3y
= 12, which is a linear equation in one variable. On solving this, you get y = 8/3. So (2, 8/3) is
another solution of 2x + 3y = 12. Similarly, choosing x = —5, you find that the equation
becomes — 10 + 3y = 12. This gives y =22/3. So, (-5, 22/3) is another solution of 2x + 3y = 12.
So there is no end to different solutions of a linear equation in two variables.
Note : An easy way of getting a solution is to take x = 0 and get the corresponding value of y.
Similarly, we can put y =0 and obtain the corresponding value of x.
GRAPH OF LINEAR EQUATIONS
The graph of an equation in x and y is the set of all points whose coordinates satisfy the
equation :
In order to draw the graph of a linear equation ax + by + c = 0 may follow the following
algorithm.
Step 1: Obtain the linear equation ax + by+ c = 0
Step 2 : Express yin terms of x i.e. y = -((ax + b)/c))
Step 3 : Put any two or three values for x and calculate the corresponding values of y from the
expression values of y from the expression obtained in step 2. Let we get points as (α1, β1), (α2,
β2), (α3, β3)
Step 4 : Plot points (α1, β1), (α2, β2), (α3, β3) on graph paper.
Step 5 : Join the points marked in step 4 to obtain. The line obtained is the graph of the
equation ax+by+c= 0
Note : (i) The reason that a degree one polynomial equation ax + by + c = 0 is called a linear
equation is that its geometrical representation is a straight line.
(ii) The graph of the equation of the form y = kx is a line which always passes through the
origin.
EQUATIONS OF LINES PARALLEL TO THE X— AXIS AND Y— AXIS
Every point on the x – axis is of the form (x, 0). The equation of the x – axis is given byy 0.
Note that y =0 can be expressed as 0. x + 1.)) 0.
Similarly, observe that the equation of they – axis is given by x = 0.
Consider the equation x -2 = 0. If this is treated as an equation in one variable x only, then it
has the unique solution x= 2, which is a point on the number line.
An equation in two variables, x -2 = 0 is represented by the line AB in the graph in below given
Note : (i) The graph ofx = a is a straight line parallel to they – axis
(ii) The graph ofy = a is a straight line parallel to thex – axis.




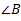
No comments